Which Path Requires
the Most Energy?
A GIF Animation
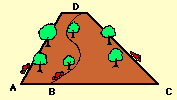Suppose that
a car traveled up three different roadways (each with varying incline
angle or slope) from the base of a mountain to the summit of the
mountain. Which path would require the most gasoline (or energy)?
Would the steepest path (path AD) require the most gasoline or would
the least steep path (path BD) require the most gasoline? Or would
each path require the same amount of gasoline?
This
situation can be simulated by use of a simple physics lab in which a
force is applied to raise a cart up an incline at constant speed to
the top of a seat. Three different incline angles could be used to
represent the three different paths up the mountain. The seat top
represents the summit of the mountain. And the amount of gasoline
(or energy) required to ascend from the base of the mountain to the
summit of the mountain would be represented by the amount of work
done on the cart to raise it from the floor to the seat top. The
amount of work done to raise the cart from the floor to the seat top
is dependent upon the force applied to the cart and the displacement
caused by this force. Typical results of such a physics lab are
depicted in the animation below.
Observe in the animation above that each path up to the seat top
(representing the summit of the mountain) requires the same amount of
work. The amount of work done by a force on any object is given by
the equation
Work = F * d *
cosine(Theta)
where F is the force,
d is the displacement and
Theta is the angle between the force and
the displacement vector.
The least steep incline (30-degree incline angle) will require
the least amount of force while the most steep incline will require
the greatest amount of force. Yet,
force is not the only variable
effecting the amount of work done by the car in ascending to a
certain elevation. Another variable is the
displacement which is caused by this
force. A look at the animation above reveals that the least steep
incline would correspond to the largest displacement and the most
steep incline would correspond to the smallest displeacement. The
final variable is Theta - the angle between the force and the
displacement vector. Theta is 0-degrees in each situation; that is,
the force is in the same direction as the displacement and thus makes
a 0-degree angle with the displacement vector. So when the force is
greatest (steep incline) the displacement is smallest and when the
force is smallest (least steep incline) the displacement is largest.
Subsequently each path happens to require the same amount of work to
elevate the object from the base to the same summit elevation.
Another perspective from which to analyze this situation is from
the perspective of potential and kinetic energy and the work-energy
theorem. The work-energy theorem states that the work done by an
external force (in this case, the force applied to the cart) changes
the total mechanical energy of the object. In fact, the amount of
work done by the applied force is equal to the total mechanical
energy change of the object. The mechanical energy of the cart takes
on two forms - kinetic energy and potential energy. In this
situation, the cart was pulled at a constant speed form a low height
to a high height. Since the speed was constant, the kinetic energy
of the cart was not changed. Only the potential energy of the cart
was changed. In each instantce (30-degree, 45-degree, and 60-degree
incline), the potential energy change of the cart was the same. The
same cart was elevated from the same initial height to the same final
height. If the potential energy change of each cart is the same,
then the total mechanical energy change is the same for each cart.
Finally, it can be reasoned that the work done on the cart must be
the same for each path.
For more information on physical descriptions of motion,
visit
The
Physics Classroom. Specific information is available there on the
following topics:
This page was created by
Tom
Henderson of
Glenbrook South
High School.
Comments and suggestions can be sent by e-mail to
Tom
Henderson.
A hearty thanks is due to lab assistant Carl Bobis for his
assistance with the graphics and GIF animation.
This page last updated on 1/20/98.