
How
Far Will It Skid?
A GIF Animation
Most driver's education classes teach future drivers that the
stopping distance of a skidding car is directly proportional to the
square of the speed of the car. That is a car traveling 10 mi/hr may
require 4 feet to skid to an abrupt halt; but a car going twice as
fast - 20 mi/hr - would require four times the distance - 16 feet to
skid to a stop. A doubling of the speed results in a quadrupling of
the stopping distance. A tripling of the speed would increase the
stopping distance by a factor of nine. And a quadrupling of the
speed would increase the stopping distance by a factor of 16. The
stopping distance is proportional to the square of the speed of the
vehicle.
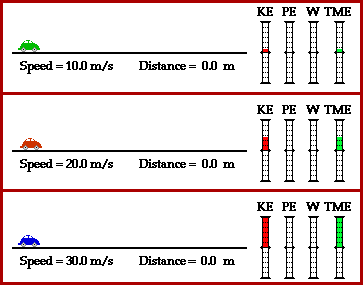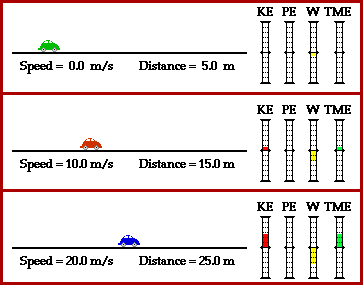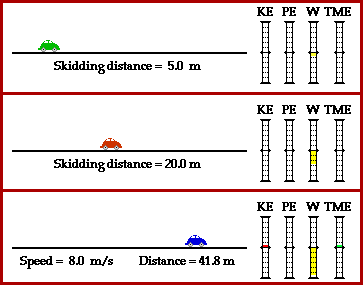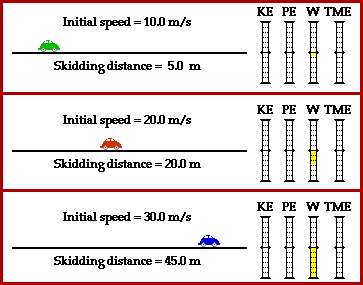
This mathematical relationship between initial speed and stopping
distance is depicted in the animation below. Three carswith
identical braking systems are traveling three different speeds.
Prior to braking, the red car is traveling twice as fast as the green
car (20 m/s is two times as big as 10 m/s); and prior to braking, the
blue car is traveling three times as fast as the green car (30 m/s is
three times as big as 10 m/s).The animation depicts that the stopping
distance of the red car is four times (22) that of the
green car and the stopping distance of the blue car is nine times
(32) that of the green car.
Perhaps you learned in a drivers education course of this
mathematical relationship between initial speed and stopping
distance. But did you know that the relationship is based upon the
physics of work and energy? Prior to braking each car has kinetic
energy (energy due to motion). The amount of kinetic energy is
dependent upon the mass and the speed of the car according to the
equation
KE = 0/5*mass*(speed)2
The blue car has the most kinetic energy since it has the
greatest speed. This is portrayed in the work-energy bar charts
above by the height of the KE bar for each car.
Once the brakes are applied, the force of friction acts upon the
car. The work done by friction on the skidding car is proportional
to stopping distance according to the equation
Work = Force * displacement * cosine(Theta)
where the displacement of the car is simply the distance the car
skids to a stop and Theta is the angle between the force and the
displacement vectors. In this case, Theta is 180 degrees since the
force of friction and the displacement of the car are in opposite
directions.
The work-energy theorem can be used to relate the work done by
friction on the car to the initial kinetic energy of the car. The
work-energy theorem is stated as an equation in the form of
KEi + PEi +
Wext = KEf + PEf
Since the potential energy of the car is the same in the initial
state (before braking) as the final state (after stopping), each term
can be cancelled from the above equation. And since the car is
finally stopped, the KEf term in the equation is zero.
Thus, the equation becomes
0.5*m*v2 + F*d*cos(180) = 0.
This equation can be rearranged so that it takes the form of
0.5*m*v2 = -F*d*cos(180)
and since the cosine(180) is -1, the equation can be re-written
as
0.5*m*v2 = F*d.
The above equation shows that the stopping distance (d) is
proportional to the square of the speed (v2). And
that's exactly what the driver's education course taught you. But
now you know: it's PHYSICS!
For more information on physical descriptions of motion,
visit
The
Physics Classroom. Specific information is available there on the
following topics:
This page was created by
Tom
Henderson of
Glenbrook South
High School.
Comments and suggestions can be sent by e-mail to
Tom
Henderson.
A hearty thanks is due to lab assistant Carl Bobis for his
assistance with the graphics and GIF animation.
This page last updated on 4/4/98.
